Working with a student who has not yet been taught simultaneous equations or 'rules' of solving equations with two unknowns is FUN - for the student as well as for the teacher...
Both of us are experiencing Aha moments in almost every session! This has been stimulating me enough to record and hence share these with teachers & parents who love reading and learning from such interactions!
So here it goes -
We had entered into the zone of equations having 2 variables in our previous session and I had realized that she is enjoying this (new) method. In fact I wanted to write on this as well, but may be after this post..
This time I had made & given her a typical problem - Ages of two people with 2 conditions which would generally propel anyone to go into simultaneous equations. And she too did so, as I had planned.
I am not mentioning the problem & the 2 equations she made out of this, because those details are not required in this post.. Will just share that x and y are the numbers in the ages of two people...
While adding and subtracting the 2 equations, we got 2 more equations:
y - x = 19 ..... (3)
She could correctly interpret this : Difference between their ages is 19.
5x + 5y = 215
Now this is where the interesting part begins:
She said,
"We divide both the sides by 25"
"Ok... And why 25?"
"...because 5x5 = 25...And we need to remove both the 5's on LHS"
...
Not at this moment, I would like you to just Pause and ask yourself as to what you would do if you find your student saying this ? Take some time and read further only after you know your answer...
...
....
....
....
....
I told her: "Okay..."
And this is what she got:
x + y = 8.6 .....(4)
....
Now at this moment, I was expecting that she would go ahead with solving equations (3) and (4) to find the values of x and y.... And finally, when we will do the Verification step, she would realize that the 2 values of x and y are not satisfying the given conditions. And probably during the investigation process, we will question the accuracy of equation (4) . But rather, another interesting thing happend:
The accuracy of this equation was questioned by her - now itself !
How?
This is what she said, while noticing the eq (4)
"Umm.... How can this be possible?"
I was surprised & curious as to what could make her wonder at this moment...
"The difference in their ages is 19... Then how can their sum be 8.6?"
".... So what? What's bothering you here?"
"Sir, in such a case, one of the ages has to be a negative number... How can that be possible?"
Aha !! Did YOU think of this aspect ? :-)
I had not thought of this. Rather, I had not even noticed this.
1) One reason could be that I was lost in the future - thinking about the possible paths of our investigation ahead because of the goof-up of Dividing by 25....
2) And another and more important reason would be - a conditioned adult mind like mine would straightaway jump into the mechanical mode of solving these 2 very-familiar equations (x+y = something and x-y = something) - without even bothering about their interpretation...
I appreciated & accepted her careful interpretation at this point (rather than arguing with her that may be the person is not born yet, so his/her age can be negative)
"So how do we go ahead now...?", I asked her curiously.
Silence for few seconds.
"What are your thoughts about the accuracy of two equations (3) and (4)?"
She checked her calculations again and confirmed that those are correct.
"So where could be the problem then?"
"I think the mistake is somewhere in Dividing by 25"
And I was delighted to hear this ... and was waiting for her next step... But I saw a roadblock at this point for sufficient time.
...
...
I would again request you to PAUSE at this moment and ask yourself as to what would YOU do at this moment ? Continue with further reading After you know your answer :)
...
....
....
....
...
So I wrote this equation 5x + 5y = 215 on the new page and asked her:
"Can we write this 5x in the addition form?"
She said it would be x+x+x+x+x and we wrote the similar one for 5y too...
"Now can you study this well and tell me what could be the value of just one set of x+y"
And she came out with 43 quite quickly. While I was happy with this.... But then surprisingly, she was Not !
"So, you mean to say that the equation x + y = 43 is not correct ?"
"it is correct...", she said with some hesitation... "But how can we get x+y = 43 by dividing 5x+5y by just 5? We should get x + y only after dividing by 25."
And while saying this she started, on the board, striking off some numbers while telling the times table of 5.
.....
You might like to PAUSE at this moment and ask yourself as to what would YOU do at this moment ? Continue with further reading After you know your answer :)
...
...
...
...
...
After giving her few seconds to think over, I drew her attention to another problem on a new page:
"Imagine I am in grade-3 and I want to calculate what is 96 / 8 and I don't even know the Long division method yet... But I know the multiplication table of 8 very well till 8 x 10 = 80 and not beyond that.... So how can you use my knowledge to help me solve this problem?"
You might like to PAUSE and think as to why I would frame and ask such a question?
....
....
...
....
...
She went ahead as I had thought saying -
"Let us see 96 as 80+16 ... we know 8 x 10 = 80 and 8 x 2=16... so the answer is 10+2 = 12" 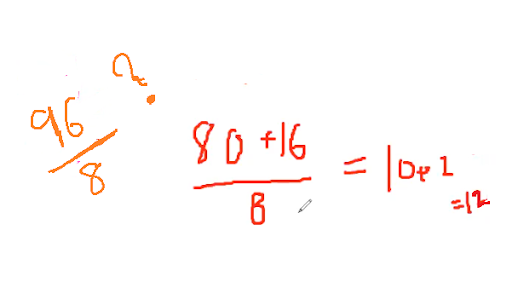
And we verified that this answer is correct because she knew that 12 x 8 = 96.
So now it was my time to play the Devil :)
"I have a doubt.... " and while saying this I started doing the same what she did some time back - striking off some numbers while telling the times table :)
"8 x 1 = 8 and 8 x 10 = 80.... So the answer is 10 + 16 = 26"
No wonder she was stunned at this :) But then she also quickly, independently & happily realized as to what I wanted to convey to her through this example...
She said that both the numbers in the top have to be divided by the same number in the bottom...
She was also satisfied with x + y = 43 now :)
But then I was not yet done... How can I miss this beautiful opportunity to make another important point ?
I asked her -
"Yes , sometimes we certainly work the way we did - striking off the numbers in the top and the bottom while telling their times table... But why did that method not work well in these problems?"
After thinking for a while, she said -
"May be it works when there are 2 numbers in the Denominator"
Again please PAUSE and think about this guess of hers... What could have made her think of such a possibility ? ;)
....
....
....
...
So she worked out this way on the board (splitting 8 as 4 + 4 in the bottom) with high hopes !
But soon she was disappointed by the result she got (24, as against the expected 12)I again gave her some time to ponder over, but since there was no response I thought it would be better idea to give her more to play with on this idea after our class... And she happily agreed to work on it by the next session...
And I am now eagerly waiting to know from her :)
1) Let me know if you too would like to know as to what happened on this matter...
2) What were your thoughts while reading this post, esp. at the instances where I suggested you to Pause and think about your strategy to move ahead ...
3) How about trying out such a conversation with your students / children ?
4) Did this post remind you of something / similar experience? Plz share...
5) What could be the possible reasons of misconceptions of the student? Have you also experienced difficulty dealing with the same topic in your class (evaluation a fraction with more terms in the top and one or more terms in the bottom)? How do you deal with that ? How well has your strategy worked?
6) Your comments about the method I adopted ?
I will be happy & thankful to you if you share your responses to (at least some of the) queries above :)
Thanks and Regards
Rupesh Gesota


