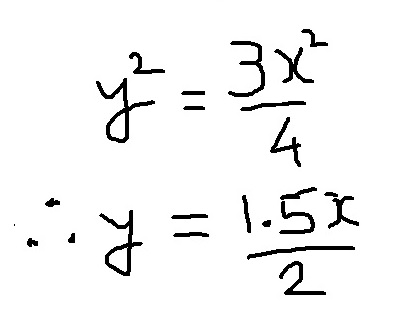So they come on the 2nd day, and I ask them -
"Could you find the value of Square root of 3?"
People who have not yet read the previous part of this post (Part-1), then it is suggested to read that first, so as to get the context of how we landed up here and what are the mistakes, conversations & learnings that happened in that process. Here is the link
http://rupeshgesota.blogspot.com/2023/02/whats-value-of-square-root-of-3-part-1.html
"Could you find the value of Square root of 3?"
"We tried.. But we didn't get"
Means?
"1.73 squared gives less than 3 and 1.74 squared gives more than 3."
I was glad that they worked with numbers having 2 digits after the decimal point, rather than just stopping at 1.7 and 1.8. But then I also wondered how come they didn't go beyond that. So I asked them -
"So what does that mean?"
"It means, value of root 3 is between 1.73 and 1.74"
"And how do you find that now?"
Puzzled look.
------
"We don't know what to do now..."
After a while, I drew their attention to the shorter first column & told them to complete this list too - write the numbers above 1.6.... They wrote till 1.1
"So these numbers are between ?"
"1 and 2"
"How did you get these numbers between 1 and 2?"
"By dividing the range into 10 parts"
"Ok.. And how did you get the numbers 1.71, 1.72, 1.73 etc.? I am asking this because I don't see these numbers in the first column?
"We knew its between 1.7 & 1.8 So we divided the range 1 to 2 into 100 parts now"
I circled around the numbers 1.7 and 1.8 when they said this and told them -
"So can we say this second column is kind of Zoomed-In-picture between 1.7 and 1.8? ... numbers which were present but not visible earlier have become visible now because you have divided the range into smaller i.e more (100) parts.. Its like you have kept a magnifying glass now on the two numbers 1.7 and 1.8", and while pointing my finger from the circled part of 1.7 and 1.8 towards the second column.
I paused a while to help them understand this new analogy being presented.
Share you thoughts after reflecting on what you read above...
...
...
"So now you say that the answer (the number) is between 1.73 and 1.74. What can we do now?"
They said - "We will divide the range further - into 1000 parts now - so that the numbers between 1.73 and 1.74 become visible" while saying this the student also circled around the pair 1.73 and 1.74
They started enlisting from 1.731, 1.732 and so on till 1.740. So I asked them what does 1.740 represent. They said its same as 1.74.
So then I told them to include another form of 1.73 too because they have circled / zoomed this number too.. Was this intervention / step needed ? why?
So in that column, he wrote 1.730 above 1.731
How about completing the first column too this way?
He checked and wrote 1.70 above 1.71
Now I drew their attention to the two circled pairs and the list of numbers next to each pair. So that they can also actually / easily see (& not just visualise) that
(1.7, 1.8) expands to range of numbers from (1.70 to 1.80) next to it, and
(1.73, 1.74) expands to range of numbers from (1.730 to 1.740) next to it.
The picture started looking like this in some time....
The squares of 1.731, 1.732 and 1.733 were calculated by them manually using std. method, but when it came to testing the squares of numbers in other columns (one with 4 and more digits after the DP), then I became their assistant and helped them getting & giving the squares of numbers which they wanted, with the help of my phone calculator.
Share your thoughts about the above paragraph (practice(s) employed by the teacher in this approach).
...
...
....
...
...
Things had gone into auto-pilot mode now and they were sort of thrilled / enjoying this process, totally surprised as this hunting never seemed to stop, against their expectation...... They said that they had never thought that square root of a number (that too such a smaller one like 3) will have so many digits :-))
I also shared with them that they don't need to enlist all the numbers in a column but can use dotted lines to indicate that.... Share why the teacher must have done so?
After some time, I stopped them and asked them what do they think about this process ?
"Sir, it seems this is never going to stop.... We are just reaching closer and closer to the answer...."
And how do you know this? (Note this question instead of validation from teacher)
"Square comes out to be 2.9999.... or 3.0000..... and few other digits are also there after 9 and 0 .... And then number of 9's and 0's keep increasing...."
So what would You do at this moment?
...
...
...
...
...
...
....
...
...
...
I asked them if they can be very sure of at least some digits in the square root of 3?
They looked for a while in all the columns and noticed the growing & unchanging section of digits. As you can see above , they have written the value of square root of 3 as 1.73205_......
I asked them if some one tells square root of 3 equals 1.732 then is it correct?
They said - "No.. Answer is closer to 1.732, but not equal to 1.732"
Share your thoughts about this response coming from students..
...
...
...
Looking at their facial expressions and body language, It was clear that this exercise was no less than an adventure ride for them :-))
So now it was Time to plug-in this correct value of root-3 into the expression they had arrived at (remember the previous post? :)
They were delighted to see that their measured lengths "almost matched" the lengths given by the formula.... Further, the value of Square root 3 was also figured out by themselves....
